problem solving simple mathematics

• Skip to main content
• Skip to primary sidebar
• Skip to footer

Additional menu

Khan Academy Blog

Free Math Worksheets — Over 100k free practice problems on Khan Academy

Looking for free math worksheets.

You’ve found something even better!

That’s because Khan Academy has over 100,000 free practice questions. And they’re even better than traditional math worksheets – more instantaneous, more interactive, and more fun!

Just choose your grade level or topic to get access to 100% free practice questions:

Kindergarten, basic geometry, pre-algebra, algebra basics, high school geometry.

• Trigonometry

Statistics and probability

High school statistics, ap®︎/college statistics, precalculus, differential calculus, integral calculus, ap®︎/college calculus ab, ap®︎/college calculus bc, multivariable calculus, differential equations, linear algebra.

• Addition and subtraction
• Place value (tens and hundreds)
• Addition and subtraction within 20
• Addition and subtraction within 100
• Addition and subtraction within 1000
• Measurement and data
• Counting and place value
• Measurement and geometry
• Place value
• Measurement, data, and geometry
• Add and subtract within 20
• Add and subtract within 100
• Add and subtract within 1,000
• Money and time
• Measurement
• Intro to multiplication
• 1-digit multiplication
• Addition, subtraction, and estimation
• Intro to division
• Understand fractions
• Equivalent fractions and comparing fractions
• More with multiplication and division
• Arithmetic patterns and problem solving
• Quadrilaterals
• Represent and interpret data
• Multiply by 1-digit numbers
• Multiply by 2-digit numbers
• Factors, multiples and patterns
• Add and subtract fractions
• Multiply fractions
• Understand decimals
• Plane figures
• Measuring angles
• Area and perimeter
• Units of measurement
• Decimal place value
• Add decimals
• Subtract decimals
• Multi-digit multiplication and division
• Divide fractions
• Multiply decimals
• Divide decimals
• Powers of ten
• Coordinate plane
• Algebraic thinking
• Converting units of measure
• Properties of shapes
• Ratios, rates, & percentages
• Arithmetic operations
• Negative numbers
• Properties of numbers
• Variables & expressions
• Equations & inequalities introduction
• Data and statistics
• Negative numbers: addition and subtraction
• Negative numbers: multiplication and division
• Fractions, decimals, & percentages
• Rates & proportional relationships
• Expressions, equations, & inequalities
• Numbers and operations
• Solving equations with one unknown
• Linear equations and functions
• Systems of equations
• Geometric transformations
• Data and modeling
• Volume and surface area
• Pythagorean theorem
• Transformations, congruence, and similarity
• Arithmetic properties
• Factors and multiples
• Reading and interpreting data
• Negative numbers and coordinate plane
• Ratios, rates, proportions
• Equations, expressions, and inequalities
• Exponents, radicals, and scientific notation
• Foundations
• Algebraic expressions
• Linear equations and inequalities
• Graphing lines and slope
• Expressions with exponents
• Quadratics and polynomials
• Equations and geometry
• Algebra foundations
• Solving equations & inequalities
• Working with units
• Linear equations & graphs
• Forms of linear equations
• Inequalities (systems & graphs)
• Absolute value & piecewise functions
• Exponents & radicals
• Exponential growth & decay
• Quadratics: Multiplying & factoring
• Quadratic functions & equations
• Irrational numbers
• Performing transformations
• Transformation properties and proofs
• Right triangles & trigonometry
• Non-right triangles & trigonometry (Advanced)
• Analytic geometry
• Conic sections
• Solid geometry
• Polynomial arithmetic
• Complex numbers
• Polynomial factorization
• Polynomial division
• Polynomial graphs
• Rational exponents and radicals
• Exponential models
• Transformations of functions
• Rational functions
• Trigonometric functions
• Non-right triangles & trigonometry
• Trigonometric equations and identities
• Analyzing categorical data
• Displaying and comparing quantitative data
• Summarizing quantitative data
• Modeling data distributions
• Exploring bivariate numerical data
• Study design
• Probability
• Counting, permutations, and combinations
• Random variables
• Sampling distributions
• Confidence intervals
• Significance tests (hypothesis testing)
• Two-sample inference for the difference between groups
• Inference for categorical data (chi-square tests)
• Advanced regression (inference and transforming)
• Analysis of variance (ANOVA)
• Scatterplots
• Data distributions
• Two-way tables
• Binomial probability
• Normal distributions
• Displaying and describing quantitative data
• Inference comparing two groups or populations
• Chi-square tests for categorical data
• More on regression
• Prepare for the 2020 AP®︎ Statistics Exam
• AP®︎ Statistics Standards mappings
• Polynomials
• Composite functions
• Probability and combinatorics
• Limits and continuity
• Derivatives: definition and basic rules
• Derivatives: chain rule and other advanced topics
• Applications of derivatives
• Analyzing functions
• Parametric equations, polar coordinates, and vector-valued functions
• Applications of integrals
• Differentiation: definition and basic derivative rules
• Differentiation: composite, implicit, and inverse functions
• Contextual applications of differentiation
• Applying derivatives to analyze functions
• Integration and accumulation of change
• Applications of integration
• AP Calculus AB solved free response questions from past exams
• AP®︎ Calculus AB Standards mappings
• Infinite sequences and series
• AP Calculus BC solved exams
• AP®︎ Calculus BC Standards mappings
• Integrals review
• Integration techniques
• Thinking about multivariable functions
• Derivatives of multivariable functions
• Applications of multivariable derivatives
• Integrating multivariable functions
• Green’s, Stokes’, and the divergence theorems
• First order differential equations
• Second order linear equations
• Laplace transform
• Vectors and spaces
• Matrix transformations
• Alternate coordinate systems (bases)

Frequently Asked Questions about Khan Academy and Math Worksheets

Why is khan academy even better than traditional math worksheets.

Khan Academy’s 100,000+ free practice questions give instant feedback, don’t need to be graded, and don’t require a printer.

What do Khan Academy’s interactive math worksheets look like?

Here’s an example:

What are teachers saying about Khan Academy’s interactive math worksheets?

“My students love Khan Academy because they can immediately learn from their mistakes, unlike traditional worksheets.”

Is Khan Academy free?

Khan Academy’s practice questions are 100% free—with no ads or subscriptions.

What do Khan Academy’s interactive math worksheets cover?

Our 100,000+ practice questions cover every math topic from arithmetic to calculus, as well as ELA, Science, Social Studies, and more.

Is Khan Academy a company?

Khan Academy is a nonprofit with a mission to provide a free, world-class education to anyone, anywhere.

Want to get even more out of Khan Academy?

Then be sure to check out our teacher tools . They’ll help you assign the perfect practice for each student from our full math curriculum and track your students’ progress across the year. Plus, they’re also 100% free — with no subscriptions and no ads.

Get Khanmigo

The best way to learn and teach with AI is here. Ace the school year with our AI-powered guide, Khanmigo. 

For learners     For teachers     For parents

Reset password New user? Sign up

Existing user? Log in

• Number Theory
• Probability

Everyday Math

• Classical Mechanics
• Electricity and Magnetism
• Computer Science
• Quantitative Finance

Take a guided, problem-solving based approach to learning Everyday Math. These compilations provide unique perspectives and applications you won't find anywhere else.

Mathematical Thinking

What's inside.

• Introduction
• Logical Reasoning
• Numerical Reasoning
• Contextual Reasoning
• Algebraic Reasoning
• Geometric Reasoning

Measurement

• Pythagoras' Geometry
• Surface Area

Community Wiki

Browse through thousands of Everyday Math wikis written by our community of experts.

Pattern Recognition

• Identifying Patterns
• Identifying Pattern Relationships
• What Comes Next?
• Finding Terms in a Sequence
• Recognizing Visual Patterns
• Irrelevant Information
• Trial and Error
• Checking Cases
• Counter-Examples
• SAT Reasoning Perfect Score
• Mental Math Tricks
• Subtracting Integers
• Rational Exponents
• Order of Operations
• Representation on the Real Line
• Natural Numbers
• Complex Fractions
• Componendo and Dividendo
• Continued Fractions
• Converting Decimals and Fractions
• Converting Fractions into Decimals
• Place Value
• Scientific Notation
• Percentages
• Converting Decimals and Percentages
• Converting Percentages and Fractions
• Ratio and Proportion
• Direct Variation
• Inverse Variation
• Speed, Distance, and Time
• Parallel Lines (Geometry)
• Area of a Triangle
• Area of a Rectangle
• Composite Figures
• Length and Area Problem Solving

Problem Loading...

Note Loading...

Set Loading...

Become a Problem-solving School

Or search by topic

Number and algebra.

• Properties of numbers
• Place value and the number system
• Calculations and numerical methods
• Fractions, decimals, percentages, ratio and proportion
• Patterns, sequences and structure
• Coordinates, functions and graphs
• Algebraic expressions, equations and formulae

Geometry and measure

• Measuring and calculating with units
• Angles, polygons, and geometrical proof
• 3D geometry, shape and space
• Transformations and constructions
• Pythagoras and trigonometry
• Vectors and matrices

Probability and statistics

• Handling, processing and representing data
• Probability

Working mathematically

• Thinking mathematically
• Mathematical mindsets

Advanced mathematics

• Decision mathematics and combinatorics
• Advanced probability and statistics

For younger learners

• Early years foundation stage

A Guide to Problem Solving

When confronted with a problem, in which the solution is not clear, you need to be a skilled problem-solver to know how to proceed. When you look at STEP problems for the first time, it may seem like this problem-solving skill is out of your reach, but like any skill, you can improve your problem-solving with practice. How do I become a better problem-solver? First and foremost, the best way to become better at problem-solving is to try solving lots of problems! If you are preparing for STEP, it makes sense that some of these problems should be STEP questions, but to start off with it's worth spending time looking at problems from other sources. This collection of NRICH problems  is designed for younger students, but it's very worthwhile having a go at a few to practise the problem-solving technique in a context where the mathematics should be straightforward to you. Then as you become a more confident problem-solver you can try more past STEP questions. One student who worked with NRICH said: "From personal experience, I was disastrous at STEP to start with. Yet as I persisted with it for a long time it eventually started to click - 'it' referring to being able to solve problems much more easily. This happens because your brain starts to recognise that problems fall into various categories and you subconsciously remember successes and pitfalls of previous 'similar' problems." A Problem-solving Heuristic for STEP Below you will find some questions you can ask yourself while you are solving a problem. The questions are divided into four phases, based loosely on those found in George Pólya's 1945 book "How to Solve It". Understanding the problem

• What area of mathematics is this?
• What exactly am I being asked to do?
• What do I know?
• What do I need to find out?
• What am I uncertain about?
• Can I put the problem into my own words?

Devising a plan

• Work out the first few steps before leaping in!
• Have I seen something like it before?
• Is there a diagram I could draw to help?
• Is there another way of representing?
• Would it be useful to try some suitable numbers first?
• Is there some notation that will help?

Carrying out the plan STUCK!

• Try special cases or a simpler problem
• Work backwards
• Guess and check
• Be systematic
• Work towards subgoals
• Imagine your way through the problem
• Has the plan failed? Know when it's time to abandon the plan and move on.

Looking back

• Have I answered the question?
• Sanity check for sense and consistency
• Check the problem has been fully solved
• Read through the solution and check the flow of the logic.

Throughout the problem solving process it's important to keep an eye on how you're feeling and making sure you're in control:

• Am I getting stressed?
• Is my plan working?
• Am I spending too long on this?
• Could I move on to something else and come back to this later?
• Am I focussing on the problem?
• Is my work becoming chaotic, do I need to slow down, go back and tidy up?
• Do I need to STOP, PEN DOWN, THINK?

Finally, don't forget that STEP questions are designed to take at least 30-45 minutes to solve, and to start with they will take you longer than that. As a last resort, read the solution, but not until you have spent a long time just thinking about the problem, making notes, trying things out and looking at resources that can help you. If you do end up reading the solution, then come back to the same problem a few days or weeks later to have another go at it.

MathPapa Practice

MathPapa Practice has practice problems to help you learn algebra.

Basic Arithmetic

Subtraction, multiplication, basic arithmetic review, multi-digit arithmetic, addition (2-digit), subtraction (2-digit), multiplication (2-digit by 1-digit), division (2-digit answer), multiplication (2-digit by 2-digit), multi-digit division, negative numbers, addition: negative numbers, subtraction: negative numbers, multiplication: negative numbers, division: negative numbers, order of operations, order of operations 1, basic equations, equations: fill in the blank 1, equations: fill in the blank 2, equations: fill in the blank 3 (order of operations), fractions of measurements, fractions of measurements 2, adding fractions, subtracting fractions, adding fractions: fill in the blank, multiplication: fractions 1, multiplication: fractions 2, division: fractions 1, division: fractions 2, division: fractions 3, addition (decimals), subtraction (decimals), multiplication 2 (example problem: 3.5*8), multiplication 3 (example problem: 0.3*80), division (decimals), division (decimals 2), percentages, percentages 1, percentages 2, chain reaction, balance arithmetic, number balance, basic balance 1, basic balance 2, basic balance 3, basic balance 4, basic balance 5, basic algebra, basic algebra 1, basic algebra 2, basic algebra 3, basic algebra 4, basic algebra 5, algebra: basic fractions 1, algebra: basic fractions 2, algebra: basic fractions 3, algebra: basic fractions 4, algebra: basic fractions 5.

• Mathematicians
• Math Lessons
• Square Roots
• Math Calculators

Simple Algebra Problems – Easy Exercises with Solutions for Beginners

JUMP TO TOPIC

Understanding Algebraic Expressions

Breaking down algebra problems, solving algebraic equations, tackling algebra word problems, types of algebraic equations, algebra for different grades.

For instance, solving the equation (3x = 7) for (x) helps us understand how to isolate the variable to find its value.

I always find it fascinating how algebra serves as the foundation for more advanced topics in mathematics and science. Starting with basic problems such as ( $(x-1)^2 = [4\sqrt{(x-4)}]^2$ ) allows us to grasp key concepts and build the skills necessary for tackling more complex challenges.

So whether you’re refreshing your algebra skills or just beginning to explore this mathematical language, let’s dive into some examples and solutions to demystify the subject. Trust me, with a bit of practice, you’ll see algebra not just as a series of problems, but as a powerful tool that helps us solve everyday puzzles.

Simple Algebra Problems and Strategies

When I approach simple algebra problems, one of the first things I do is identify the variable.

The variable is like a placeholder for a number that I’m trying to find—a mystery I’m keen to solve. Typically represented by letters like ( x ) or ( y ), variables allow me to translate real-world situations into algebraic expressions and equations.

An algebraic expression is a mathematical phrase that can contain ordinary numbers, variables (like ( x ) or ( y )), and operators (like add, subtract, multiply, and divide). For example, ( 4x + 7 ) is an algebraic expression where ( x ) is the variable and the numbers ( 4 ) and ( 7 ) are terms. It’s important to manipulate these properly to maintain the equation’s balance.

Solving algebra problems often starts with simplifying expressions. Here’s a simple method to follow:

• Combine like terms : Terms that have the same variable can be combined. For instance, ( 3x + 4x = 7x ).
• Isolate the variable : Move the variable to one side of the equation. If the equation is ( 2x + 5 = 13 ), my job is to get ( x ) by itself by subtracting ( 5 ) from both sides, giving me ( 2x = 8 ).

With algebraic equations, the goal is to solve for the variable by performing the same operation on both sides. Here’s a table with an example:

Algebra word problems require translating sentences into equations. If a word problem says “I have six less than twice the number of apples than Bob,” and Bob has ( b ) apples, then I’d write the expression as ( 2b – 6 ).

Understanding these strategies helps me tackle basic algebra problems efficiently. Remember, practice makes perfect, and each problem is an opportunity to improve.

In algebra, we encounter a variety of equation types and each serves a unique role in problem-solving. Here, I’ll brief you about some typical forms.

Linear Equations : These are the simplest form, where the highest power of the variable is one. They take the general form ( ax + b = 0 ), where ( a ) and ( b ) are constants, and ( x ) is the variable. For example, ( 2x + 3 = 0 ) is a linear equation.

Polynomial Equations : Unlike for linear equations, polynomial equations can have variables raised to higher powers. The general form of a polynomial equation is ( $a_nx^n + a_{n-1}x^{n-1} + … + a_2x^2 + a_1x + a_0 = 0$ ). In this equation, ( n ) is the highest power, and ( $a_n$ ), ( $a_{n-1} $), …, ( $a_0$ ) represent the coefficients which can be any real number.

• Binomial Equations : They are a specific type of polynomial where there are exactly two terms. Like ($ x^2 – 4 $), which is also the difference of squares, a common format encountered in factoring.

To understand how equations can be solved by factoring, consider the quadratic equation ( $x^2$ – 5x + 6 = 0 ). I can factor this into ( (x-2)(x-3) = 0 ), which allows me to find the roots of the equation.

Here’s how some equations look when classified by degree:

Remember, identification and proper handling of these equations are essential in algebra as they form the basis for complex problem-solving.

In my experience with algebra, I’ve found that the journey begins as early as the 6th grade, where students get their first taste of this fascinating subject with the introduction of variables representing an unknown quantity.

I’ve created worksheets and activities aimed specifically at making this early transition engaging and educational.

6th Grade :

Moving forward, the complexity of algebraic problems increases:

7th and 8th Grades :

• Mastery of negative numbers: students practice operations like ( -3 – 4 ) or ( -5 $\times$ 2 ).
• Exploring the rules of basic arithmetic operations with negative numbers.
• Worksheets often contain numeric and literal expressions that help solidify their concepts.

Advanced topics like linear algebra are typically reserved for higher education. However, the solid foundation set in these early grades is crucial. I’ve developed materials to encourage students to understand and enjoy algebra’s logic and structure.

Remember, algebra is a tool that helps us quantify and solve problems, both numerical and abstract. My goal is to make learning these concepts, from numbers to numeric operations, as accessible as possible, while always maintaining a friendly approach to education.

I’ve walked through various simple algebra problems to help establish a foundational understanding of algebraic concepts. Through practice, you’ll find that these problems become more intuitive, allowing you to tackle more complex equations with confidence.

Remember, the key steps in solving any algebra problem include:

• Identifying variables and what they represent.
• Setting up the equation that reflects the problem statement.
• Applying algebraic rules such as the distributive property ($a(b + c) = ab + ac$), combining like terms, and inverse operations.
• Checking your solutions by substituting them back into the original equations to ensure they work.

As you continue to engage with algebra, consistently revisiting these steps will deepen your understanding and increase your proficiency. Don’t get discouraged by mistakes; they’re an important part of the learning process.

I hope that the straightforward problems I’ve presented have made algebra feel more manageable and a little less daunting. Happy solving!

• Pre Calculus
• Probability
• Sets & Set Theory
• Trigonometry

Math Problem Solving Strategies

In these lessons, we will learn some math problem solving strategies for example, Verbal Model (or Logical Reasoning), Algebraic Model, Block Model (or Singapore Math), Guess & Check Model and Find a Pattern Model.

Related Pages Solving Word Problems Using Block Models Heuristic Approach to Problem-Solving Algebra Lessons

Problem Solving Strategies

The strategies used in solving word problems:

• What do you know?
• What do you need to know?
• Draw a diagram/picture

Solution Strategies Label Variables Verbal Model or Logical Reasoning Algebraic Model - Translate Verbal Model to Algebraic Model Solve and Check.

Solving Word Problems

Step 1: Identify (What is being asked?) Step 2: Strategize Step 3: Write the equation(s) Step 4: Answer the question Step 5: Check

Problem Solving Strategy: Guess And Check

Using the guess and check problem solving strategy to help solve math word problems.

Example: Jamie spent $40 for an outfit. She paid for the items using $10, $5 and $1 bills. If she gave the clerk 10 bills in all, how many of each bill did she use?

Problem Solving : Make A Table And Look For A Pattern

• Identify - What is the question?
• Plan - What strategy will I use to solve the problem?
• Solve - Carry out your plan.
• Verify - Does my answer make sense?

Example: Marcus ran a lemonade stand for 5 days. On the first day, he made $5. Every day after that he made $2 more than the previous day. How much money did Marcus made in all after 5 days?

Find A Pattern Model (Intermediate)

In this lesson, we will look at some intermediate examples of Find a Pattern method of problem-solving strategy.

Example: The figure shows a series of rectangles where each rectangle is bounded by 10 dots. a) How many dots are required for 7 rectangles? b) If the figure has 73 dots, how many rectangles would there be?

a) The number of dots required for 7 rectangles is 52.

b) If the figure has 73 dots, there would be 10 rectangles.

Example: Each triangle in the figure below has 3 dots. Study the pattern and find the number of dots for 7 layers of triangles.

The number of dots for 7 layers of triangles is 36.

Example: The table below shows numbers placed into groups I, II, III, IV, V and VI. In which groups would the following numbers belong? a) 25 b) 46 c) 269

Solution: The pattern is: The remainder when the number is divided by 6 determines the group. a) 25 ÷ 6 = 4 remainder 1 (Group I) b) 46 ÷ 6 = 7 remainder 4 (Group IV) c) 269 ÷ 6 = 44 remainder 5 (Group V)

Example: The following figures were formed using matchsticks.

a) Based on the above series of figures, complete the table below.

b) How many triangles are there if the figure in the series has 9 squares?

c) How many matchsticks would be used in the figure in the series with 11 squares?

b) The pattern is +2 for each additional square.   18 + 2 = 20   If the figure in the series has 9 squares, there would be 20 triangles.

c) The pattern is + 7 for each additional square   61 + (3 x 7) = 82   If the figure in the series has 11 squares, there would be 82 matchsticks.

Example: Seven ex-schoolmates had a gathering. Each one of them shook hands with all others once. How many handshakes were there?

Total = 6 + 5 + 4 + 3 + 2 + 1 = 21 handshakes.

The following video shows more examples of using problem solving strategies and models. Question 1: Approximate your average speed given some information Question 2: The table shows the number of seats in each of the first four rows in an auditorium. The remaining ten rows follow the same pattern. Find the number of seats in the last row. Question 3: You are hanging three pictures in the wall of your home that is 16 feet wide. The width of your pictures are 2, 3 and 4 feet. You want space between your pictures to be the same and the space to the left and right to be 6 inches more than between the pictures. How would you place the pictures?

The following are some other examples of problem solving strategies.

Explore it/Act it/Try it (EAT) Method (Basic) Explore it/Act it/Try it (EAT) Method (Intermediate) Explore it/Act it/Try it (EAT) Method (Advanced)

Finding A Pattern (Basic) Finding A Pattern (Intermediate) Finding A Pattern (Advanced)

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving 

Katie Keeton

Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.  

Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.

This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations. 

What are problem-solving strategies?

Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies: 

• Draw a model
• Use different approaches
• Check the inverse to make sure the answer is correct

Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better. 

Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques

Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.

20 Math Strategies For Problem-Solving

Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem. 

Here are 20 strategies to help students develop their problem-solving skills. 

Strategies to understand the problem

Strategies that help students understand the problem before solving it helps ensure they understand: 

• The context
• What the key information is
• How to form a plan to solve it

Following these steps leads students to the correct solution and makes the math word problem easier .

Here are five strategies to help students understand the content of the problem and identify key information. 

1. Read the problem aloud

Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.

2. Highlight keywords 

When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed.  For example, if the word problem asks how many are left, the problem likely requires subtraction.  Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.

3. Summarize the information

Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary.  Summaries should include only the important information and be in simple terms that help contextualize the problem.

4. Determine the unknown

A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer.  Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.

5. Make a plan

Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it.  The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer.  Encourage students to make a list of each step they need to take to solve the problem before getting started.

Strategies for solving the problem 

1. draw a model or diagram.

Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process.  It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.

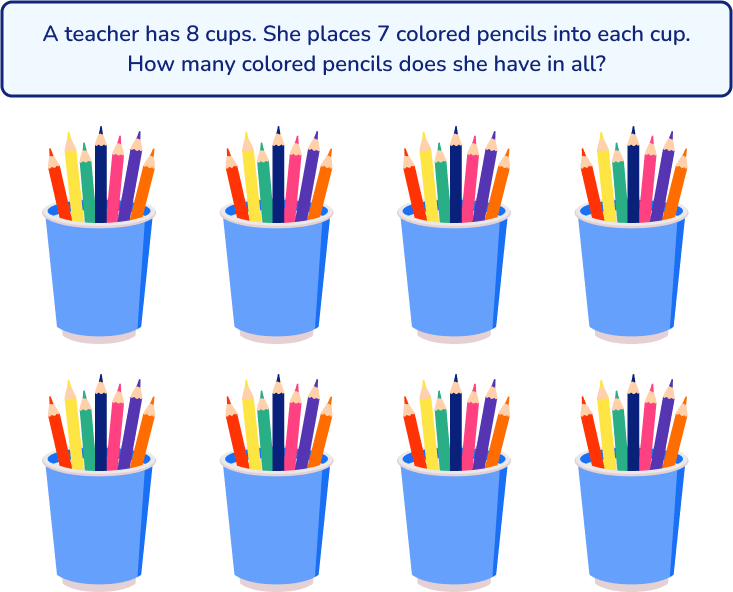
Similarly, you could draw a model to represent the objects in the problem:

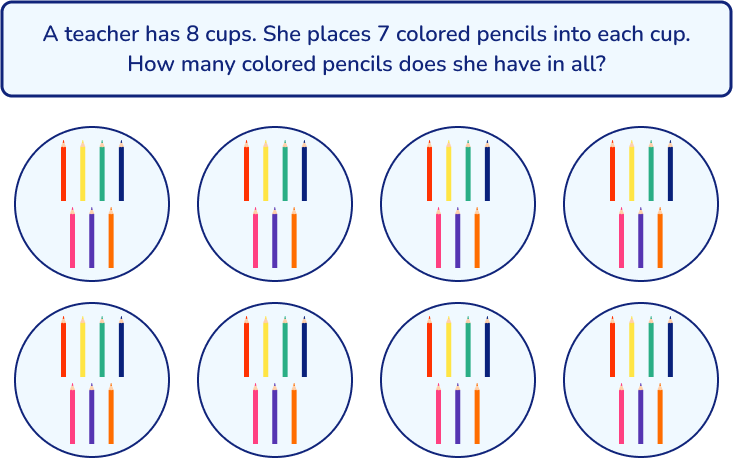
2. Act it out

This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives .  When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts.  The examples below show how 1st-grade students could “act out” an addition and subtraction problem:

3. Work backwards

Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution.  This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.

For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71.  Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.

4. Write a number sentence

When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved.  It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.

5. Use a formula

Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve.   Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.

Strategies for checking the solution 

Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense. 

There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.

Here are five strategies to help students check their solutions. 

1. Use the Inverse Operation

For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work. 

2. Estimate to check for reasonableness

Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable.  Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten.  For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10.  When the estimate is clear the two numbers are close. This means your answer is reasonable. 

3. Plug-In Method

This method is particularly useful for algebraic equations. Specifically when working with variables.  To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓

4. Peer Review

Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly.   Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills.  If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.

5. Use a Calculator

A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.  

Step-by-step problem-solving processes for your classroom

In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems. 

Polya’s 4 steps include:

• Understand the problem
• Devise a plan
• Carry out the plan

Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall. 

Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom. 

Here are 5 problem-solving strategies to introduce to students and use in the classroom.

How Third Space Learning improves problem-solving 

Resources .

Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking. 

Explore the range of problem solving resources for 2nd to 8th grade students. 

One-on-one tutoring 

Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards. 

Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice. 

Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.

Problem-solving

Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra. 

Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.

READ MORE :

• 8 Common Core math examples
• Tier 3 Interventions: A School Leaders Guide
• Tier 2 Interventions: A School Leaders Guide
• Tier 1 Interventions: A School Leaders Guide

There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model  • act it out  • work backwards  • write a number sentence • use a formula

Here are 10 strategies for problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model  • Act it out  • Work backwards  • Write a number sentence • Use a formula

1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back

Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.

Related articles

Retrieval Practice: A Foolproof Method To Improve Student Retention and Recall

5 Tried And Tested Strategies To Increase Student Engagement

Rote Memorization: Is It Effective In Education?

5 Effective Instructional Strategies Educators Can Use In Every Classroom

Ultimate Guide to Metacognition [FREE]

Looking for a summary on metacognition in relation to math teaching and learning?

Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.

Privacy Overview

Solving Equations

What is an equation.

An equation says that two things are equal. It will have an equals sign "=" like this:

That equations says:

what is on the left (x − 2)  equals  what is on the right (4)

So an equation is like a statement " this equals that "

What is a Solution?

A Solution is a value we can put in place of a variable (such as x ) that makes the equation true .

Example: x − 2 = 4

When we put 6 in place of x we get:

which is true

So x = 6 is a solution.

How about other values for x ?

• For x=5 we get "5−2=4" which is not true , so x=5 is not a solution .
• For x=9 we get "9−2=4" which is not true , so x=9 is not a solution .

In this case x = 6 is the only solution.

You might like to practice solving some animated equations .

More Than One Solution

There can be more than one solution.

Example: (x−3)(x−2) = 0

When x is 3 we get:

(3−3)(3−2) = 0 × 1 = 0

And when x is 2 we get:

(2−3)(2−2) = (−1) × 0 = 0

which is also true

So the solutions are:

x = 3 , or x = 2

When we gather all solutions together it is called a Solution Set

The above solution set is: {2, 3}

Solutions Everywhere!

Some equations are true for all allowed values and are then called Identities

Example: sin(−θ) = −sin(θ) is one of the Trigonometric Identities

Let's try θ = 30°:

sin(−30°) = −0.5 and

−sin(30°) = −0.5

So it is true for θ = 30°

Let's try θ = 90°:

sin(−90°) = −1 and

−sin(90°) = −1

So it is also true for θ = 90°

Is it true for all values of θ ? Try some values for yourself!

How to Solve an Equation

There is no "one perfect way" to solve all equations.

A Useful Goal

But we often get success when our goal is to end up with:

x = something

In other words, we want to move everything except "x" (or whatever name the variable has) over to the right hand side.

Example: Solve 3x−6 = 9

Now we have x = something ,

and a short calculation reveals that x = 5

Like a Puzzle

In fact, solving an equation is just like solving a puzzle. And like puzzles, there are things we can (and cannot) do.

Here are some things we can do:

• Add or Subtract the same value from both sides
• Clear out any fractions by Multiplying every term by the bottom parts
• Divide every term by the same nonzero value
• Combine Like Terms
• Expanding (the opposite of factoring) may also help
• Recognizing a pattern, such as the difference of squares
• Sometimes we can apply a function to both sides (e.g. square both sides)

Example: Solve √(x/2) = 3

And the more "tricks" and techniques you learn the better you will get.

Special Equations

There are special ways of solving some types of equations. Learn how to ...

• solve Quadratic Equations
• solve Radical Equations
• solve Equations with Sine, Cosine and Tangent

Check Your Solutions

You should always check that your "solution" really is a solution.

How To Check

Take the solution(s) and put them in the original equation to see if they really work.

Example: solve for x:

2x x − 3 + 3 = 6 x − 3     (x≠3)

We have said x≠3 to avoid a division by zero.

Let's multiply through by (x − 3) :

2x + 3(x−3) = 6

Bring the 6 to the left:

2x + 3(x−3) − 6 = 0

Expand and solve:

2x + 3x − 9 − 6 = 0

5x − 15 = 0

5(x − 3) = 0

Which can be solved by having x=3

Let us check x=3 using the original question:

2 × 3 3 − 3 + 3  =   6 3 − 3

Hang On: 3 − 3 = 0 That means dividing by Zero!

And anyway, we said at the top that x≠3 , so ...

x = 3 does not actually work, and so:

There is No Solution!

That was interesting ... we thought we had found a solution, but when we looked back at the question we found it wasn't allowed!

This gives us a moral lesson:

"Solving" only gives us possible solutions, they need to be checked!

• Note down where an expression is not defined (due to a division by zero, the square root of a negative number, or some other reason)
• Show all the steps , so it can be checked later (by you or someone else)

• Solve equations and inequalities
• Simplify expressions
• Factor polynomials
• Graph equations and inequalities
• Advanced solvers
• All solvers
• Arithmetics
• Determinant
• Percentages
• Scientific Notation
• Inequalities

What can QuickMath do?

QuickMath will automatically answer the most common problems in algebra, equations and calculus faced by high-school and college students.

• The algebra section allows you to expand, factor or simplify virtually any expression you choose. It also has commands for splitting fractions into partial fractions, combining several fractions into one and cancelling common factors within a fraction.
• The equations section lets you solve an equation or system of equations. You can usually find the exact answer or, if necessary, a numerical answer to almost any accuracy you require.
• The inequalities section lets you solve an inequality or a system of inequalities for a single variable. You can also plot inequalities in two variables.
• The calculus section will carry out differentiation as well as definite and indefinite integration.
• The matrices section contains commands for the arithmetic manipulation of matrices.
• The graphs section contains commands for plotting equations and inequalities.
• The numbers section has a percentages command for explaining the most common types of percentage problems and a section for dealing with scientific notation.

Math Topics

More solvers.

• Add Fractions
• Simplify Fractions

Over 5 Billion Problems Solved

Step-by-step examples.

• Adding Using Long Addition
• Long Subtraction
• Long Multiplication
• Long Division
• Dividing Using Partial Quotients Division
• Converting Regular to Scientific Notation
• Arranging a List in Order
• Expanded Notation
• Prime or Composite
• Comparing Expressions
• Converting to a Percentage
• Finding the Additive Inverse
• Finding the Multiplicative Inverse
• Reducing Fractions
• Finding the Reciprocal
• Converting to a Decimal
• Converting to a Mixed Number
• Adding Fractions
• Subtracting Fractions
• Multiplying Fractions
• Dividing Fractions
• Converting Ratios to Fractions
• Converting Percents to Decimal
• Converting Percents to Fractions
• Converting the Percent Grade to Degree
• Converting the Degree to Percent Grade
• Finding the Area of a Rectangle
• Finding the Perimeter of a Rectangle
• Finding the Area of a Square
• Finding the Perimeter of a Square
• Finding the Area of a Circle
• Finding the Circumference of a Circle
• Finding the Area of a Triangle
• Finding the Area of a Trapezoid
• Finding the Volume of a Box
• Finding the Volume of a Cylinder
• Finding the Volume of a Cone
• Finding the Volume of a Pyramid
• Finding the Volume of a Sphere
• Finding the Surface Area of a Box
• Finding the Surface Area of a Cylinder
• Finding the Surface Area of a Cone
• Finding the Surface Area of a Pyramid
• Converting to a Fraction
• Simple Exponents
• Prime Factorizations
• Finding the Factors
• Simplifying Fractions
• Converting Grams to Kilograms
• Converting Grams to Pounds
• Converting Grams to Ounces
• Converting Feet to Inches
• Converting to Meters
• Converting Feet to Miles
• Converting Feet to Yards
• Converting to Feet
• Converting to Yards
• Converting Miles to Feet
• Converting Miles to Kilometers
• Converting Miles to Yards
• Converting Kilometers to Miles
• Converting Kilometers to Meters
• Converting Meters to Feet
• Converting Meters to Inches
• Converting Ounces to Grams
• Converting Ounces to Pounds
• Converting Ounces to Tons
• Converting Pounds to Grams
• Converting Pounds to Ounces
• Converting Pounds to Tons
• Converting Yards to Feet
• Converting Yards to Millimeters
• Converting Yards to Inches
• Converting Yards to Miles
• Converting Yards to Meters
• Converting Fahrenheit to Celsius
• Converting Celsius to Fahrenheit
• Finding the Median
• Finding the Mean (Arithmetic)
• Finding the Mode
• Finding the Minimum
• Finding the Maximum
• Finding the Lower or First Quartile
• Finding the Upper or Third Quartile
• Finding the Five Number Summary
• Finding a Point's Quadrant
• Finding the Midpoint of a Line Segment
• Distance Formula
• Arithmetic Operations
• Combining Like Terms
• Determining if the Expression is a Polynomial
• Distributive Property
• Simplifying
• Multiplication
• Polynomial Addition
• Polynomial Subtraction
• Polynomial Multiplication
• Polynomial Division
• Simplifying Expressions
• Evaluate the Expression Using the Given Values
• Multiplying Polynomials Using FOIL
• Identifying Degree
• Operations on Polynomials
• Negative Exponents
• Evaluating Radicals
• Solving by Adding/Subtracting
• Solving by Multiplying/Dividing
• Solving Containing Decimals
• Solving for a Variable
• Solving Linear Equations
• Solving Linear Inequalities
• Finding the Quadratic Constant of Variation
• Converting the Percent Grade to Slope
• Converting the Slope to Percent Grade
• Finding Equations Using Slope-Intercept
• Finding the Slope
• Finding the y Intercept
• Calculating Slope and y-Intercept
• Rewriting in Slope-Intercept Form
• Finding Equations Using the Slope-Intercept Formula
• Finding Equations Using Two Points
• Finding a Perpendicular Line Containing a Given Point
• Finding a Parallel Line Containing a Given Point
• Finding a Parallel Line to the Given Line
• Finding a Perpendicular Line to the Given Line
• Finding Ordered Pair Solutions
• Using a Table of Values to Graph an Equation
• Finding the Equation Using Point-Slope Form
• Finding the Surface Area of a Sphere
• Solving by Graphing
• Finding the LCM of a List of Expressions
• Finding the LCD of a List of Expressions
• Determining if the Number is a Perfect Square
• Finding the Domain
• Evaluating the Difference Quotient
• Solving Using the Square Root Property
• Determining if True
• Finding the Holes in a Graph
• Finding the Common Factors
• Expand a Trinomial with the Trinomial Theorem
• Finding the Start Point Given the Mid and End Points
• Finding the End Point Given the Start and Mid Points
• Finding the Slope and y-Intercept
• Finding the Equation of the Parabola
• Finding the Average Rate of Change
• Finding the Slope of the Perpendicular Line to the Line Through the Two Points
• Rewriting Using Negative Exponents
• Synthetic Division
• Maximum Number of Real Roots/Zeros
• Finding All Possible Roots/Zeros (RRT)
• Finding All Roots with Rational Root Test (RRT)
• Finding the Remainder
• Finding the Remainder Using Long Polynomial Division
• Reordering the Polynomial in Ascending Order
• Reordering the Polynomial in Descending Order
• Finding the Leading Term
• Finding the Leading Coefficient
• Finding the Degree, Leading Term, and Leading Coefficient
• Finding the GCF of a Polynomial
• Factoring Out Greatest Common Factor (GCF)
• Identifying the Common Factors
• Cancelling the Common Factors
• Finding the LCM using GCF
• Finding the GCF
• Factoring Trinomials
• Trinomial Squares
• Factoring Using Any Method
• Factoring a Difference of Squares
• Factoring a Sum of Cubes
• Factoring by Grouping
• Factoring a Difference of Cubes
• Determine if an Expression is a Factor
• Determining if Factor Using Synthetic Division
• Find the Factors Using the Factor Theorem
• Determining if Polynomial is Prime
• Determining if the Polynomial is a Perfect Square
• Expand using the Binomial Theorem
• Factoring over the Complex Numbers
• Finding All Integers k Such That the Trinomial Can Be Factored
• Determining if Linear
• Rewriting in Standard Form
• Finding x and y Intercepts
• Finding Equations Using the Point Slope Formula
• Finding Equations Given Point and y-Intercept
• Finding the Constant Using Slope
• Finding the Slope of a Parallel Line
• Finding the Slope of a Perpendicular Line
• Simplifying Absolute Value Expressions
• Solving with Absolute Values
• Finding the Vertex for the Absolute Value
• Rewriting the Absolute Value as Piecewise
• Calculating the Square Root
• Simplifying Radical Expressions
• Rationalizing Radical Expressions
• Solving Radical Equations
• Rewriting with Rational (Fractional) Exponents
• Finding the Square Root End Point
• Operations on Rational Expressions
• Determining if the Point is a Solution
• Solving over the Interval
• Finding the Range
• Finding the Domain and Range
• Solving Rational Equations
• Adding Rational Expressions
• Subtracting Rational Expressions
• Multiplying Rational Expressions
• Finding the Equation Given the Roots
• Finding the Asymptotes
• Finding the Constant of Variation
• Finding the Equation of Variation
• Substitution Method
• Addition/Elimination Method
• Graphing Method
• Determining Parallel Lines
• Determining Perpendicular Lines
• Dependent, Independent, and Inconsistent Systems
• Finding the Intersection (and)
• Using the Simplex Method for Constraint Maximization
• Using the Simplex Method for Constraint Minimization
• Finding the Union (or)
• Finding the Equation with Real Coefficients
• Solving in Terms of the Arbitrary Variable
• Finding a Direct Variation Equation
• Finding the Slope for Every Equation
• Finding a Variable Using the Constant of Variation
• Quadratic Formula
• Solving by Factoring
• Solve by Completing the Square
• Finding the Perfect Square Trinomial
• Finding the Quadratic Equation Given the Solution Set
• Finding a,b, and c in the Standard Form
• Finding the Discriminant
• Finding the Zeros by Completing the Square
• Quadratic Inequalities
• Rational Inequalities
• Converting from Interval to Inequality
• Converting to Interval Notation
• Rewriting as a Single Interval
• Determining if the Relation is a Function
• Finding the Domain and Range of the Relation
• Finding the Inverse of the Relation
• Finding the Inverse
• Determining if One Relation is the Inverse of Another
• Determining if Surjective (Onto)
• Determining if Bijective (One-to-One)
• Determining if Injective (One to One)
• Rewriting as an Equation
• Rewriting as y=mx+b
• Solving Function Systems
• Find the Behavior (Leading Coefficient Test)
• Determining Odd and Even Functions
• Describing the Transformation
• Finding the Symmetry
• Arithmetic of Functions
• Domain of Composite Functions
• Finding Roots Using the Factor Theorem
• Determine if Injective (One to One)
• Determine if Surjective (Onto)
• Finding the Vertex
• Finding the Sum
• Finding the Difference
• Finding the Product
• Finding the Quotient
• Finding the Domain of the Sum of the Functions
• Finding the Domain of the Difference of the Functions
• Finding the Domain of the Product of the Functions
• Finding the Domain of the Quotient of the Functions
• Finding Roots (Zeros)
• Identifying Zeros and Their Multiplicities
• Finding the Bounds of the Zeros
• Proving a Root is on the Interval
• Finding Maximum Number of Real Roots
• Function Composition
• Rewriting as a Function
• Determining if a Function is Rational
• Determining if a Function is Proper or Improper
• Maximum/Minimum of Quadratic Functions
• Finding All Complex Number Solutions
• Rationalizing with Complex Conjugates
• Vector Arithmetic
• Finding the Complex Conjugate
• Finding the Magnitude of a Complex Number
• Simplifying Logarithmic Expressions
• Expanding Logarithmic Expressions
• Evaluating Logarithms
• Rewriting in Exponential Form
• Converting to Logarithmic Form
• Exponential Expressions
• Exponential Equations
• Converting to Radical Form
• Find the Nth Root of the Given Value
• Simplifying Matrices
• Finding the Variables
• Solving the System of Equations Using an Inverse Matrix
• Finding the Dimensions
• Multiplication by a Scalar
• Subtraction
• Finding the Determinant of the Resulting Matrix
• Finding the Inverse of the Resulting Matrix
• Finding the Identity Matrix
• Finding the Scalar multiplied by the Identity Matrix
• Simplifying the Matrix Operation
• Finding the Determinant of a 2x2 Matrix
• Finding the Determinant of a 3x3 Matrix
• Finding the Determinant of Large Matrices
• Inverse of a 2x2 Matrix
• Inverse of an nxn Matrix
• Finding Reduced Row Echelon Form
• Finding the Transpose
• Finding the Adjoint
• Finding the Cofactor Matrix
• Finding the Pivot Positions and Pivot Columns
• Finding the Basis and Dimension for the Row Space of the Matrix
• Finding the Basis and Dimension for the Column Space of the Matrix
• Finding the LU Decomposition of a Matrix
• Identifying Conic Sections
• Identifying Circles
• Finding a Circle Using the Center and Another Point
• Finding a Circle by the Diameter End Points
• Finding the Parabola Equation Using the Vertex and Another Point
• Finding the Properties of the Parabola
• Finding the Vertex Form of the Parabola
• Finding the Vertex Form of an Ellipse
• Finding the Vertex Form of a Circle
• Finding the Vertex Form of a Hyperbola
• Finding the Standard Form of a Parabola
• Finding the Expanded Form of an Ellipse
• Finding the Expanded Form of a Circle
• Finding the Expanded Form of a Hyperbola
• Vector Addition
• Vector Subtraction
• Vector Multiplication by a Scalar
• Finding the Length
• Finding the Position Vector
• Determining Column Spaces
• Finding an Orthonormal Basis by Gram-Schmidt Method
• Rewrite the System as a Vector Equality
• Finding the Rank
• Finding the Nullity
• Finding the Distance
• Finding the Plane Parallel to a Line Given four 3d Points
• Finding the Intersection of the Line Perpendicular to Plane 1 Through the Origin and Plane 2
• Finding the Eigenvalues
• Finding the Characteristic Equation
• Finding the Eigenvectors/Eigenspace of a Matrix
• Proving a Transformation is Linear
• Finding the Kernel of a Transformation
• Projecting Using a Transformation
• Finding the Pre-Image
• Finding the Intersection of Sets
• Finding the Union of Number Sets
• Determining if a Set is a Subset of Another Set
• Determining if Two Sets are Mutually Exclusive
• Finding the Set Complement of Two Sets
• Finding the Power Set
• Finding the Cardinality
• Finding the Cartesian Product of Two Sets
• Determining if a Set is a Proper Subset of Another Set
• Finding the Function Rule
• Finding the Square or Rectangle Area Given Four Points
• Finding the Square or Rectangle Perimeter Given Four Points
• Finding the Square or Rectangle Area Given Three Points
• Finding the Square or Rectangle Perimeter Given Three Points
• Finding the Equation of a Circle
• Finding the Equation of a Hyperbola
• Finding the Equation of an Ellipse
• Partial Fraction Decomposition
• Finding an Angle Using another Angle
• Pythagorean Theorem
• Finding the Sine
• Finding the Cosine
• Finding the Tangent
• Finding the Trig Value
• Converting to Degrees, Minutes, and Seconds
• Finding Trig Functions Using Identities
• Finding Trig Functions Using the Right Triangle
• Converting Radians to Degrees
• Converting Degrees to Radians
• Finding a Reference Angle
• Finding a Supplement
• Finding a Complement
• Converting RPM to Radians per Second
• Finding the Quadrant of the Angle
• Graphing Sine & Cosine Functions
• Graphing Other Trigonometric Functions
• Amplitude, Period, and Phase Shift
• Finding the Other Trig Values in a Quadrant
• Finding the Exact Value
• Finding the Value Using the Unit Circle
• Expanding Trigonometric Expressions
• Expanding Using Double-Angle Formulas
• Expanding Using Triple-Angle Formulas
• Expanding Using Sum/Difference Formulas
• Simplify Using Pythagorean Identities
• Simplify by Converting to Sine/Cosine
• Inverting Trigonometric Expressions
• Finding the Trig Value of an Angle
• Expanding Using De Moivre's Theorem
• Verifying Trigonometric Identities
• Using Fundamental Identities
• Solving Standard Angle Equations
• Complex Trigonometric Equations
• Solving the Triangle
• Find the Roots of a Complex Number
• Complex Operations
• Trigonometric Form of a Complex Number
• Converting to Polar Coordinates
• Identifying and Graphing Circles
• Identifying and Graphing Limacons
• Identifying and Graphing Roses
• Identifying and Graphing Cardioids
• Difference Quotient
• Finding Upper and Lower Bounds
• Evaluating Functions
• Right Triangle Trigonometry
• Arithmetic Sequences/Progressions
• Geometric Sequences/Progressions
• Finding the Next Term of the Sequence
• Finding the nth Term Given a List of Numbers
• Finding the nth Term
• Finding the Sum of First n Terms
• Expanding Series Notation
• Finding the Sum of the Series
• Finding the Sum of the Infinite Geometric Series
• Converting to Rectangular Coordinates
• Evaluating Limits Approaching a Value
• Evaluating Limits Approaching Infinity
• Finding the Angle Between the Vectors
• Determining if the Point is on the Graph
• Finding the Antiderivative
• Checking if Continuous Over an Interval
• Determining if a Series is Divergent
• Using the Integral Test for Convergence
• Determining if an Infinite Series is Convergent Using Cauchy's Root Test
• Using the Limit Definition to Find the Tangent Line at a Given Point
• Finding the nth Derivative
• Finding the Derivative Using Product Rule
• Finding the Derivative Using Quotient Rule
• Finding the Derivative Using Chain Rule
• Use Logarithmic Differentiation to Find the Derivative
• Finding the Derivative
• Implicit Differentiation
• Using the Limit Definition to Find the Derivative
• Evaluating the Derivative
• Finding Where dy/dx is Equal to Zero
• Finding the Linearization
• Finding a Tangent Line to a Curve
• Checking if Differentiable Over an Interval
• The Mean Value Theorem
• Finding the Inflection Points
• Find Where the Function Increases/Decreases
• Finding the Critical Points of a Function
• Find Horizontal Tangent Line
• Evaluating Limits with L'Hospital Rule
• Local Maxima and Minima
• Finding the Absolute Maximum and Minimum on the Given Interval
• Finding Concavity using the Second Derivative
• Finding the Derivative using the Fundamental Theorem of Calculus
• Find the Turning Points
• Finding the Integral
• Evaluating Definite Integrals
• Evaluating Indefinite Integrals
• Substitution Rule
• Finding the Arc Length
• Finding the Average Value of the Derivative
• Finding the Average Value of the Equation
• Finding Area Between Curves
• Finding the Volume
• Finding the Average Value of the Function
• Finding the Root Mean Square
• Integration by Parts
• Trigonometric Integrals
• Trigonometric Substitution
• Integration by Partial Fractions
• Eliminating the Parameter from the Function
• Verify the Solution of a Differential Equation
• Solve for a Constant Given an Initial Condition
• Find an Exact Solution to the Differential Equation
• Verify the Existence and Uniqueness of Solutions for the Differential Equation
• Solve for a Constant in a Given Solution
• Solve the Bernoulli Differential Equation
• Solve the Linear Differential Equation
• Solve the Homogeneous Differential Equation
• Solve the Exact Differential Equation
• Approximate a Differential Equation Using Euler's Method
• Finding Elasticity of Demand
• Finding the Consumer Surplus
• Finding the Producer Surplus
• Finding the Gini Index
• Finding the Geometric Mean
• Finding the Quadratic Mean (RMS)
• Find the Mean Absolute Deviation
• Finding the Mid-Range (Mid-Extreme)
• Finding the Interquartile Range (H-Spread)
• Finding the Midhinge
• Finding the Standard Deviation
• Finding the Skew of a Data Set
• Finding the Range of a Data Set
• Finding the Variance of a Data Set
• Finding the Class Width
• Solving Combinations
• Solving Permutations
• Finding the Probability of Both Independent Events
• Finding the Probability of Both Dependent Events
• Finding the Probability for Both Mutually Exclusive Events
• Finding the Conditional Probability for Independent Events
• Determining if Given Events are Independent/Dependent Events
• Determining if Given Events are Mutually Exclusive Events
• Finding the Probability of Both not Mutually Exclusive Events
• Finding the Conditional Probability Using Bayes' Theorem
• Finding the Probability of the Complement
• Describing Distribution's Two Properties
• Finding the Expectation
• Finding the Variance
• Finding the Probability of a Binomial Distribution
• Finding the Probability of the Binomial Event
• Finding the Mean
• Finding the Relative Frequency
• Finding the Percentage Frequency
• Finding the Upper and Lower Class Limits of the Frequency Table
• Finding the Class Boundaries of the Frequency Table
• Finding the Class Width of the Frequency Table
• Finding the Midpoints of the Frequency Table
• Finding the Mean of the Frequency Table
• Finding the Variance of the Frequency Table
• Finding the Standard Deviation of the Frequency Table
• Finding the Cumulative Frequency of the Frequency Table
• Finding the Relative Frequency of the Frequency Table
• Finding the Median Class Interval of the Frequency Table
• Finding the Modal Class of the Frequency Table
• Creating a Grouped Frequency Distribution Table
• Finding the Data Range
• Finding a z-Score for a Normal Distribution
• Approximating Using Normal Distribution
• Finding the Probability of the z-Score Range
• Finding the Probability of a Range in a Nonstandard Normal Distribution
• Finding the z-Score Using the Table
• Finding the z-Score
• Testing the Claim
• Finding a t-Value for a Confidence Level
• Finding the Critical t-Value
• Setting the Alternative Hypothesis
• Setting the Null Hypothesis
• Determining if Left, Right, or Two Tailed Test Given the Null Hypothesis
• Determining if Left, Right, or Two Tailed Test Given the Alternative Hypothesis
• Finding Standard Error
• Finding the Linear Correlation Coefficient
• Determining if the Correlation is Significant
• Finding a Regression Line
• Cramer's Rule
• Solving using Matrices by Elimination
• Solving using Matrices by Row Operations
• Solving using an Augmented Matrix
• Finding the Simple Interest Received
• Finding the Present Value with Compound Interest
• Finding the Simple Interest Future Value
• Finding the Future Value with Continuous Interest
• Finding the Norm in Real Vector Space
• Finding the Direction Angle of the Vector
• Finding the Cross Product of Vectors
• Finding the Dot Product of Vectors
• Determining if Vectors are Orthogonal
• Finding the Distance Between the Vectors
• Finding a Unit Vector in the Same Direction as the Given Vector
• Finding the Angle Between Two Vectors Using the Cross Product
• Finding the Angle Between Two Vectors Using the Dot Product
• Finding the Projection of One Vector Onto another Vector
• Matrices Addition
• Matrices Subtraction
• Matrices Multiplication
• Finding the Trace
• Finding the Basis
• Matrix Dimension
• Convert to a Linear System
• Diagonalizing a Matrix
• Determining the value of k for which the system has no solutions
• Linear Independence of Real Vector Spaces
• Finding the Null Space
• Determining if the Vector is in the Span of the Set
• Finding the Number of Protons
• Finding the Number of Electrons
• Finding the Number of Neutrons
• Finding the Mass of a Single Atom
• Finding the Electron Configuration
• Finding the Atomic Mass
• Finding the Atomic Number
• Finding the Mass Percentages
• Finding Oxidation Numbers
• Balancing Chemical Equations
• Balancing Burning Reactions
• Finding the Density at STP
• Determining if the Compound is Soluble in Water
• Finding Mass
• Finding Density
• Finding Weight
• Finding Force
• Finding the Work Done
• Finding Angular Velocity
• Finding Centripetal Acceleration
• Finding Final Velocity
• Finding Average Acceleration
• Finding Displacement
• Finding Voltage Using the Ohm's Law
• Finding Electrical Power
• Finding Kinetic Energy
• Finding Power
• Finding Wavelength
• Finding Frequency
• Finding Pressure of the Gas

Popular Problems

• Pre-Algebra
• Trigonometry
• Precalculus
• Finite Math
• Linear Algebra
• Recent Popular Problems

Calculator Pages

Formula pages.

• Algebra Formulas
• Trigonometry Formulas
• Geometric Formulas
• Terms ( Premium )
• DO NOT SELL MY INFO
• Mathway © 2024

Please ensure that your password is at least 8 characters and contains each of the following:

• a special character: @$#!%*?&

• Member Login
• Pre-Algebra Lessons
• Pre-Algebra Word Problems
• Pre-Algebra Calculators
• Algebra Lessons
• Algebra Word Problems
• Algebra Proofs
• Advanced Algebra
• Algebra Calculators
• Geometry Lessons
• Geometry Word Problems
• Geometry Proofs
• Geometry Calculators
• Trigonometry Lessons
• Numeration System
• Basic Concepts of Set Theory
• Consumer Math
• Baseball Math
• Math for Nurses
• Statistics Made Easy
• High School Physics
• Basic Mathematics Store
• Math Vocabulary Quizzes
• SAT Math Prep
• Math Skills by Grade Level
• Ask an Expert
• Other Websites
• K-12 Worksheets
• Worksheets Generator
• Algebra Worksheets
• Geometry Worksheets
• Fun Online Math Games
• Math Puzzles
• Math Tricks

Basic Math Word Problems

You encounter and solve basic math word problems on a daily basis without thinking about it. Knowing how to tackle and solve word problems is an important skill in school. 

🧮 Learn to Tackle some Important Pre-Algebra Word Problems

🧠 general problem solving.

Learn effective approaches to tackle various math problems, improving your overall problem-solving skills.

🔢 Whole Number Word Problems

Practice applying addition in real-world scenarios to strengthen your problem-solving abilities.

Enhance your subtraction skills by working through practical, everyday situations.

Develop a deeper understanding of multiplication by solving problems in context.

Improve your division skills with problems that relate to real-life situations.

Learn to analyze and solve problems involving comparisons between quantities.

Strengthen your understanding of place value through practical problem-solving.

Explore how Venn diagrams can be used to solve problems involving sets and relationships.

Practice calculating and interpreting averages in various real-world contexts.

🍕 Fraction Word Problems

Improve your overall understanding of fractions through diverse problem-solving scenarios.

Practice adding fractions in practical contexts to reinforce your skills.

Enhance your ability to subtract fractions by solving real-world problems.

Develop a deeper understanding of fraction multiplication through practical applications.

Strengthen your skills in dividing fractions with problems set in everyday situations.

⚖️ Ratio and Proportion Word Problems

Learn to solve problems involving proportions, a crucial skill in many real-world applications.

Practice using ratios to solve problems, enhancing your understanding of relative quantities.

💯 Percentage Word Problems

Improve your skills in calculating and interpreting percentages in various scenarios.

Learn how to determine and interpret percent error, an important concept in many fields.

🔄 Conversions

Practice converting between different units of area, a useful skill in various practical situations.

As you try to understand the basic math word problems presented here, you will become more aware of these problems and sharpen your basic math skills at the same time.

In the end, you should feel comfortable solving most word problems that use basic math as a skill!

Try to follow this unit in the order given here so you can get the most benefit. However, it is not necessary to understand any of the lessons.

Algebra word problems

Have A Great Basic Math Word Problem?

Share it here with a very detailed solution!

Enter Your Title

Add a Picture/Graphic Caption (optional)  

Click here to upload more images (optional)

Author Information (optional)

To receive credit as the author, enter your information below.

Submit Your Contribution

• Check box to agree to these   submission guidelines .
• I am at least 16 years of age.
• I understand and accept the privacy policy .
• I understand that you will display my submission on your website.

(You can preview and edit on the next page)

What Other Visitors Have Said

Click below to see contributions from other visitors to this page...

Click here to write your own.

Special Math Topics

Applied math.

About me :: Privacy policy :: Disclaimer :: Donate   Careers in mathematics  

Copyright © 2008-2021. Basic-mathematics.com. All right reserved

Build Strong Math Vocabulary Skills Using These Simple Strategies

Learning new vocabulary is a fundamental part of understanding math concepts. Use these strategies to build both fluency and engagement.

Your content has been saved!

Math class doesn’t seem like the most obvious choice for word walls, glossary lists, and word of the day games. But a strong understanding of math terms is essential for mastering concepts—meaning strategies for building robust vocabulary are surprisingly useful. 

Recently, fifth-grade math teacher Kathleen Palmieri began to wonder how well her students understood the complex terms used in textbooks and word problems. So she performed some action research by pulling out 10 key terms—including exponent, base, equivalent, and estimate—and asked students to define those terms using words or numbers, she writes in a recent piece for MiddleWeb . About 40 percent of her kids could write a basic definition, exposing significant gaps in conceptual fluency.

“What I discovered in my students’ responses was that learning math terminology is more than studying a list of words,” she said, concluding that regular practice with new math terminology facilitates mathematical discourse and understanding. “It is much more of an architecture of learning where concepts need to be explored and a pathway of understanding needs to be blazed before a mathematical term can be attached to establish true meaning.”

Here are a few of the ways Palmieri and other teachers “immerse students in the language of math”—while keeping student engagement high. 

1. Let students do the defining: Students need to contextualize words before they can understand them, and need repeated exposure to them before they sink in. 

As an alternative to Palmieri’s baseline assessment, have students pull out the key terms they think will be important later. Literacy specialist Rebecca Alber asked her students to skim a chapter from a textbook and identify their own vocabulary list . Students would then rate each term by whether they “know it,” “sort of know it,” or “don't know it at all.” Afterward, they wrote out a definition or took their best guess at the term’s meaning.

“Before they turn in these pre-reading charts, be sure to emphasize this is not about ‘being right,’” she advised. “They are providing you with information to guide next steps in class vocabulary instruction.”

Similarly, Palmieri provides some introductory context and asks students to add to an expanding glossary. Research has largely dispelled the practice of writing out memorized definitions from textbooks, so Palmieri takes a different tack. Students take an active role in coming up with definitions based on their learning. As students learn more about the terms and how they’re used, they update definitions. At the end of the lesson, to consolidate learning, it may prove helpful to review all the terms as a class.

2. Get creative with word walls: Instead of writing terms on a word wall and hanging it up for students to glance at, Palmieri has students write terms on colorful Post-Its and affix them to bulletin boards. She also gets great engagement from letting students use art supplies to creatively show what they’ve learned: “Bubble letters, examples of problems and definitions with graphics are truly fun ‘math’ activities,” she explains. “Students present and explain their term and then proudly display their poster in the classroom.” 

Unlike static word walls, these strategies involve principles of constructivism , an active and social learning theory where learners build on previous knowledge and create new learning themselves. As students learn new concepts, they can define terms in real-time, make adjustments as the concepts deepen, and hang them around the classroom for others to learn from.

3. Make it a game: Math instruction doesn’t have to be drab, says Palmieri. You can introduce familiar word games like Pictionary, where students draw out clues and others try to guess the concept. She also plays a game called “What’s My Term?” where “students verbally give clues as others listen.” 

Likewise, language specialist and Harvard lecturer Rebecca Rolland suggests a game where students show they know what terms mean by listing “non-examples” of things they are studying. For instance, acute angles can look “‘sharp’ but not ‘curvy’ or ‘wavy’ or ‘square,’” she says. Ask students to come up with creative non-examples and explain their thought processes. “That same acute angle might look like a door that’s partly shut, but not like a smile or a cloud.” 

Still others play match games with index cards face down on a table, or encourage students to create definitions that rhyme or fit to music. In these cases, the game itself is perhaps less important than the act of engaging students to commit the terms to memory. 

4. Word(s) of the day: To reinforce specific concepts, Palmieri has the class come up with a word of the day or week, depending on the duration of the lesson. Students count how often the word is used and in which contexts (e.g., in word problems, during class discussion, in small group activities). 

Inspired by research she had done that suggested students need to use a word between six and 30 times to truly learn it, sixth-grade teacher Megan Kelly began picking three words to focus on for a day and reviewing the terms at the start of class. During class, she emphasizes the words herself, and asks students to use the words as many times as they can with a partner. 

“I used the words a ton in my directions and made a big deal whenever I heard a student say one of our goal words,” she says. “Everyone wants to be in on the fun, so each time I praised someone for using the word, there was an increase in others using them too.”

5. Break down word problems: Word problems are notoriously difficult, especially when challenging language obscures the intent of the question. Before students solve word problems numerically, Palmieri has the whole class perform a close read for sense-making. Together they pull out key words and create a written response. Working out word problems as a group is a well-established strategy. Teachers at Concourse Village Elementary School in New York City use a 3-read protocol : First, they read the word problem aloud to the class without numbers, then students read the complete problem on their own and pull out key terms before reading it together as a class. The 3-read protocol clarifies “what they’re reading and helps to build their fluency,” says Blair Pacheco, a teacher who has used the strategy with her students.

The Maths — No Problem! 2025 Whakawhitinga Edition

Because New Zealand's learners deserve extraordinary.

→ powerful., → award-winning., → unmistakably new zealand..

Daily information sessions. Monday - Friday 3:30pm (NZDT)

Adapted to meet the goals of Te Mātaiaho and the Te Tiriti o Waitangi Principles.

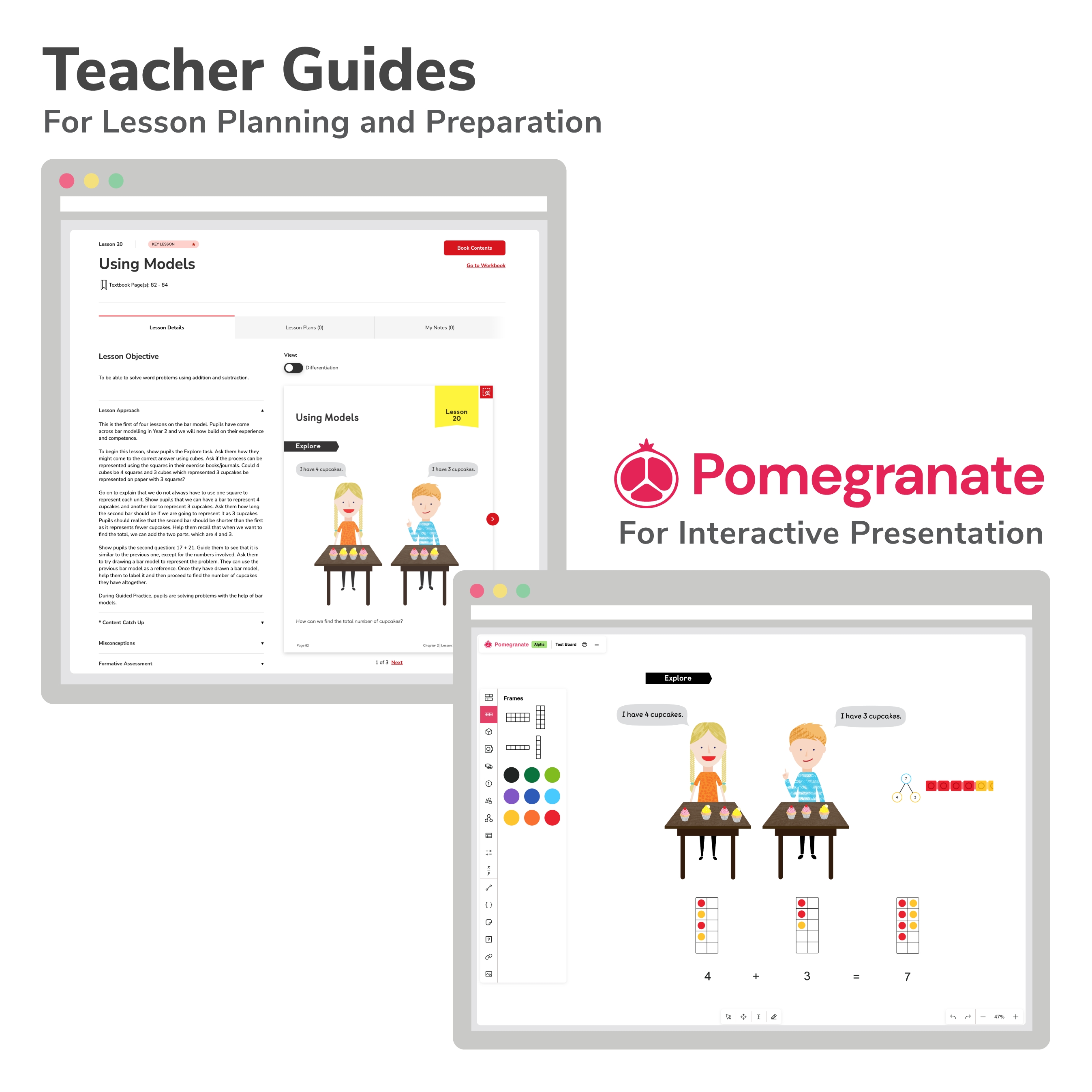
For Teachers

Essential teaching tools:

Comprehensive Teacher Guides streamline lesson planning, while Bridging Guides ensure smooth level transitions.

The interactive presentation tools within Pomegranate captivate students with dynamic visuals, empowering educators to deliver impactful mathematics instruction with confidence and ease.

The 28 video courses in the Fundamentals Online Course are designed to help teachers understand how to teach with the Maths — No Problem! series.

For Students

Essential learning resources :

Engaging Textbooks present concepts clearly and reinforce learning, while practice-oriented Workbooks offer opportunities to apply knowledge through carefully varied questions that build relational understanding.

For younger children:

Maths Reading Books introduce concepts through engaging stories. Workbook Journals combine exercises with reflective writing, encouraging thought expression, and progress tracking.

Redefining excellence in mathematics, one classroom at a time.

Created with teachers in mind, because we believe every educator deserves the tools to inspire mathematical brilliance with confidence and ease.

Equipped with professional-grade resources and cutting-edge instruments, you can make a real difference for all the children in your school while you confidently bridge the transition to the new Te Mātaiaho, launching in January 2025.

Because every educator deserves cutting-edge tools to inspire mathematical brilliance with assurance and simplicity.

Your Teacher Guides instil confidence by providing you with credible and comprehensive details to deliver effective lessons to the whole class with conviction every time.

→   Masterfully Crafted by Experienced Experts

→   Clear,   Consistent and Coherent Guidance

→   Efficiency-Bolstering Plans and Particulars

Your Bridging Guides illuminate the path to the new curriculum, providing you with crystal­-clear direction for every step of the journey.

→ Clear, Step-By-Step Directions

→ Instructions and Guidance

→ Navigate by Curriculum Objectives or by Lessons

Partner with the Maths — No Problem! community for its proven track record of enhancing student achievement and benefit from a commitment to teacher satisfaction.

→ Crafted for you   — the Teachers

→ Trusted by Millions of Children Worldwide

→ Backed by Evidence, Built on Research

How It Works

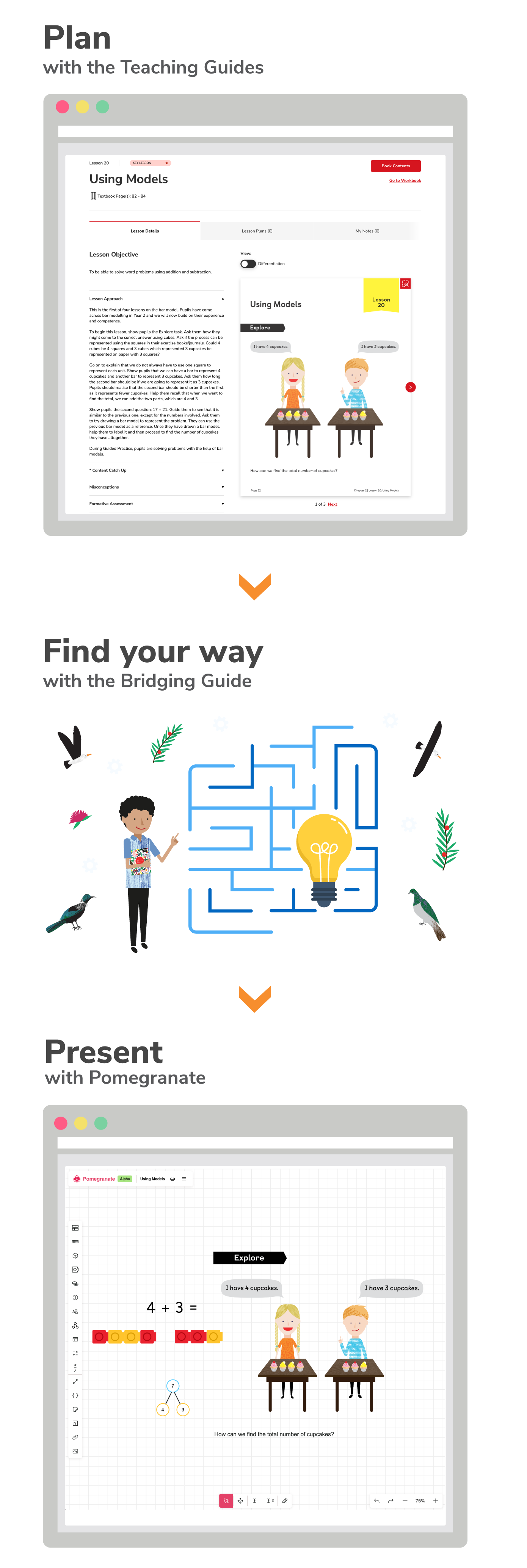
1. Teacher Guides

Confident teachers teach brilliantly, support for educators reimagined.

With intuitive planning and streamlined guidance, the Teacher Guides support you with effortless lesson preparation. The guides include innovative pedagogy with cutting-edge teaching methods to inspire strong learning. Assistance is integrated into the guides for seamless support and a fluid teaching experience. Access formative assessment tools for real-time insight into student understanding to transform your practice.

Streamlined lesson planning: Guides for each lesson provide essential planning information, teaching approaches, and solutions to common misconceptions — includes variation techniques, curriculum alignment, and suggested resources.

Formative assessment: Tools to evaluate student understanding and guide lesson progression.

Teacher empowerment: Carefully designed guides boost confidence in delivering effective maths instruction.

Comprehensive support: Access worked answers for all questions and a concise Getting Started guide for efficient Teacher Guide navigation.

Fundamentals course: A 28-video series offering insights into Maths — No Problem! methodology, enhancing educators' ability to deliver effective maths lessons.

Parent Section: Videos for parents that you can put on your school website.

Parent Resources: Worked answers, downloadables, and printable worksheets (allowing books to stay at school).

2. Bridging Guides

Seamless Transitions, Limitless Potential

Effortlessly navigate curriculum changes with your intuitive bridging guide..

Switching curricula can bring significant uncertainty and confusion for both students and teachers. Your Bridging Guide is designed to illuminate the path forward, providing clarity and direction through this transition.

Addresses Content Gaps: Identifies and highlights new material, providing resources to teach it effectively.

Curriculum Transition Support: Specifically designed for the transition between the current and new New Zealand Curriculum.

Comprehensive Coverage: Maps across both current and new curricula to ensure all necessary content is covered.

Meets New Expectations: Maps all lessons to the new curriculum requirements.

Resource Navigation: Shows teachers where to find everything needed to meet new expectations.

Smooth Transition: Aims to ease the transition for both teachers and students.

3. Pomegranate: Maths, Illuminated

Simplify. engage. inspire., sleek, intuitive presentation tool that brings mathematics to life..

Transform your mathematics lessons into sophisticated, immersive experiences that spark curiosity and nurture deep understanding.

Captivating Visuals: Breathe life into mathematical concepts with elegant, vibrant graphics that effortlessly capture attention and deepen understanding.

Seamless Interaction: Encourage active learning through fluid touch interactions, intuitive gestures, and smooth concept demonstrations.

Perfect Harmony: Flawlessly integrated with our curriculum, Pomegranate creates a unified, coherent learning journey.

Tailored Experience: Effortlessly adapt presentations to your unique style, ensuring a personalized approach for every classroom.

Instant Insights: Seamlessly incorporate quick checks to gauge understanding in real-time, enabling fluid, responsive instruction.

4. Fundamentals Course

Master your craft, access clear, engaging video courses that will transform your teaching..

Unlock the full potential of Maths — No Problem with our intuitive Fundamentals course. Designed with the elegance and simplicity you expect from us, this series of 28 video lessons seamlessly guides you through our innovative approach. Transform your teaching, inspire your students, and redefine mathematics education — all at your fingertips.

Comprehensive Course: Dive into our 28-video Fundamentals course which offers a thorough exploration of the Maths — No Problem! methodology and its practical application in the classroom.

User-Friendly Format: Navigate through bite-sized video lessons with ease, designed to accommodate educators' busy schedules and varying levels of experience.

Real-World Application: Observe seasoned educators demonstrating key concepts and strategies, bridging the gap between theory and practice in mathematics instruction.

Self-Paced Learning: Tailor your professional development journey by progressing through the course at your own speed, with the flexibility to revisit content as needed.

Ongoing Resources: Access a growing library of supplementary materials and updates, ensuring your Maths — No Problem! skills remain current and effective.

Because every child deserves the best opportunities in life.

Deep conceptual understanding

Through the spiral curriculum and the emphasis on problem-solving, students develop a profound grasp of mathematical concepts rather than just memorizing procedures.

Confidence and resilience

By revisiting and expanding on topics, learners build confidence in their mathematical abilities and develop resilience when faced with challenging problems.

Critical thinking skills

The focus on real-world problem-solving and high-level reasoning nurtures students' critical thinking abilities, preparing them to be innovative thinkers beyond the maths classroom.

1. Early Years Wonder: Where Maths Begins

Years 0 and 1.

For our youngest learners, we've crafted a magical world of numbers . Our resources blend seamlessly with early learning principles, nurturing pre-numeracy skills through enchanting stories, playful adventures, and tactile explorations.

It's not just maths; it's the first step in a lifelong journey of discovery.

Foundations Picture Books: A Visual Journey into Mathematics

Embark on a mathematical adventure with James Alan Hermanson's enchanting picture book series.

Expertly crafted with input from Series Consultant Yeap Ban Har, these visually stunning books weave core mathematical concepts into captivating stories.

Perfect for young learners, they make maths exploration a magical journey.

Mathematical Concepts: The stories subtly introduce core mathematical ideas through visual cues and storytelling.

Rich Illustrations: Painstakingly crafted visuals bring the magical worlds to life, enhancing the learning experience.

Interactive Elements: The books offer opportunities for games and exploration, encouraging active engagement with mathematical concepts.

Diverse Characters: From tangram cats to brilliant bunnies, the books feature a range of charming characters that appeal to young readers.

Multi-Concept Learning: Each book covers multiple mathematical ideas, providing a comprehensive introduction to foundational maths skills.

Foundations Workbook Journals: Hands-on Practice for Mathematical Mastery

Transform mathematical concepts into practical skills through fun, thoughtfully designed exercises.

These carefully designed workbooks provide hands-on practice to reinforce the mathematical concepts introduced in the picture books.

Image-focused design: The workbooks prioritize visual elements over text to reduce cognitive load for early learners.

Deep mastery approach: Activities are developed to provide direct access to core mathematical principles.

Comprehensive coverage: Three workbook journals cover the entire school year.

Regular practice: The journals provide two activities per week.

Practical application: Suggested activities utilize equipment and real-world items found in classrooms.

Progressive content: Each workbook (A, B, and C) covers different topics, gradually introducing more complex concepts.

2. Where Mathematical Mastery Flourishes

Years 2 to 8.

Our carefully designed curriculum spirals through these formative years , transforming young minds into confident problem-solvers. With each concept revisited and expanded, students don't just learn maths — they master it.

It's not just education; it's the art of nurturing tomorrow's innovators and critical thinkers.

Textbooks: Elevate Your Learning

More than just textbooks, these are the cornerstones of a transformative mathematical experience.

Discover a world of mathematical brilliance with our meticulously crafted textbooks. Each page is an invitation to explore, innovate, and master the art of numbers.

Integrated seamlessly with our suite of learning tools, they create an ecosystem where mastery isn't just achieved — it's inevitable.

Intuitive Lessons: Sleek, streamlined content that builds understanding with precision.

Innovative Problem-Solving: Engaging challenges that spark creativity and critical thinking.

Stunning Visuals: Minimalist diagrams and models that illuminate complex concepts.

Seamless Progression: A fluid journey through topics, each revisit adding depth and nuance.

Refined Practice: Carefully curated exercises that hone skills with elegance.

Thoughtful Reflection: Insightful prompts that encourage deep, meaningful learning.

Workbooks: Refine Your Skills

More than just a companion to the textbooks — they're an essential part of the learning experience.

The independent practice section is a cornerstone of our workbooks, embodying the principle of variation. Here, students encounter:

Mathematical Variation: Problems that subtly shift in complexity and approach, deepening understanding.

Embodiment Variation : Diverse conceptual representations of mathematical concepts, fostering a profound grasp of mathematical principles.

Varied Challenges: Problems that evolve through mathematical and embodiment variation, deepening understanding.

Thoughtful Progression: A seamless flow from knowing to applying to reasoning.

Elegant Exercises: Carefully curated problems that challenge and inspire.

Minimalist Design: Clean layouts that focus attention on what matters most.

Reflective Spaces: Areas for notes and insights, encouraging metacognition.

Visual Thinking: Opportunities to sketch and model mathematical ideas.

The Maths — No Problem! Programme

Watch an in-depth video on the methodology behind the maths — no problem award-winning programme., download sample textbook and workbook lessons..

Years 0 and 1 Topics Include:

Matching, sorting, comparing, and ordering objects and numbers

Recognizing and creating simple patterns (AB, AAB, ABC)

Counting forwards and backwards

Understanding positional language

Introduction to basic operations: addition, subtraction, and concepts of halving and sharing

Exploring number bonds to 10

Using five and ten frames

Identifying odd and even numbers

Basic geometry

Measurement concepts: time, capacity, length, and mass

Introduction to data collection and representation

Year 2 Topics Include:

Numbers and Counting: Covers numbers up to 100, including counting, writing, comparing, and ordering

Addition and Subtraction: Introduces various strategies for adding and subtracting, including number bonds and word problems

Multiplication and Division: Basic concepts of skip counting, equal groups, sharing, and doubling

Fractions: Introduction to halves and quarters

Geometry: Includes recognizing shapes, patterns, and positions

Measurement: Covers length, height, time, money, volume, capacity, and mass

Problem-solving: Incorporates word problems and practical applications throughout various topics

Year 3 Topics Include:

Numbers and Counting: Counting, place value, comparing numbers, number bonds, and patterns

Addition and Subtraction: Simple adding and subtracting, renaming, and working with three numbers

Multiplication and Division: Focus on times tables, equal groups, sharing, and odd/even numbers

Measurement: Length (metres and centimetres), mass (kilograms and grams), temperature, and volume (litres and millilitres)

Money: Writing amounts, counting, comparing, calculating totals and change

Geometry: Two-dimensional and three-dimensional shapes, including sides, vertices, symmetry, and patterns

Fractions: Equal parts, naming, representing, comparing and ordering fractions

Time: Telling time to 5 minutes, durations, and sequencing events

Data Handling: Reading and interpreting graphs, and conducting investigations

Problem Solving: Word problems integrated throughout various topics

Year 4 Topics Include:

Rounding Numbers: Focuses on rounding whole numbers

Money: Includes naming amounts, showing amounts, adding and subtracting money, and calculating change

Time: Covers telling time, measuring time in seconds, minutes, and hours, and converting between units

Picture Graphs and Bar Graphs: Teaches investigating, drawing, reading and analyzing various types of graphs

Fractions: Extensive coverage including counting in tenths, equivalent fractions, comparing fractions, and solving word problems

Angles: Introduces angle concepts, identifying angles, and comparing angles

Shapes: Covers 2D and 3D shapes

Perimeter of Figures: Teaches measuring and calculating perimeters of various shapes

Year 5 Topics Include:

Numbers and Operations: Further multiplication and division, including multi-digit numbers

Graphs: Drawing, investigating, analysing and reading pictograms, bar graphs, and line graphs.

Fractions: Equivalent fractions, mixed numbers, adding and subtracting fractions

Time: 24-hour clock, time conversions, and duration problems

Decimals: Writing tenths and hundredths, comparing and ordering decimals

Money: Writing, comparing, and rounding amounts, solving money problems

Measurement: Length, mass, and volume conversions and estimations

Geometry: Angles, triangles, quadrilaterals, symmetry, and shape classification

Position and Movement: Plotting points and describing translations

Probability: Predicted frequency, theoretical and experimental probabilities

Year 6 Topics Include:

Whole Numbers: Operations with numbers up to 1,000,000, including addition, subtraction, multiplication, and division

Fractions: Improper fractions, mixed numbers, equivalent fractions, comparing and ordering fractions, and operations with fractions

Decimals: Reading, writing, comparing, and performing operations with decimals

Percentages: Writing percentages, converting between fractions, decimals, and percentages

Graphs: Reading, investigating, planning, analysing and interpreting various types of graphs, including tables and line graphs

Geometry: Types of angles, measuring angles, and drawing lines and angles

Years 7 and 8 Topics Include:

Numbers and Operations: Decimals, percentages, ratio, and negative numbers

Algebra: Patterns, algebraic expressions, formulae, and equations

Geometry: Area, perimeter, volume, angles, circles, and 3D shapes

Measurement: Converting units of length, mass, volume, and time

Statistics and Probability: Averages (mean), pie charts, and line graphs

Problem Solving: Word problems involving various mathematical concepts

Beyond the Series

Best Practice Schools

Driven by an army of best practice schools, there is a lot more support when you are a Maths — No Problem! school, including a community network for schools to help each other. Find the New Zealand schools already using Maths — No Problem!

Professional Development

We provide customized training for schools at additional cost, tailored to your staff and students' needs. Our expert trainers offer workshops, demo lessons, and collaborative planning to help teachers effectively implement Maths — No Problem! in their classrooms.

Support For Schools

Maths — No Problem! has been used by millions of children and has trained thousands of teachers. Just ask — We are here to help.

Testimonials

Does the series cover the entire te mātaiaho, the new zealand curriculum.

The series follows the goals of the Te Mātaiaho, the New Zealand curriculum, ensuring a comprehensive and enriched mathematical education.

When is the best time to start?

The best time to start is now, as there is no need to wait to implement the very best mathematics education programme. Meet with one of our representatives to start your Maths — No Problem! journey towards success.

Can Maths — No Problem! be used with composite classes?

Yes, the programme is adaptable for composite classes, offering differentiation strategies and support for teaching multiple year groups simultaneously.

Can the maths lessons still be creative and engaging?

Absolutely! Yes! Maths — No Problem! encourages creativity and student-led learning, providing a framework for exploration and interactive problem-solving.

Do Maths — No Problem! schools see improved results?

New Zealand schools using Maths — No Problem! have reported significant improvements in student achievement and engagement. The mastery approach helps build deep understanding, leading to better long-term results and increased confidence in mathematics.

How does the programme handle diverse abilities in a class?

Maths — No Problem! supports differentiation with extension activities for advanced learners and additional support for those who need it. This allows all students to progress at their own pace while working on the same core concepts.

Can Maths — No Problem! be used alongside other resources?

While comprehensive, many schools use the Maths — No Problem! programme as their core maths curriculum and supplement it with additional resources as needed.

How does Maths — No Problem! support schools implementing the approach?

Maths — No Problem! provides comprehensive professional development through workshops, webinars, and ongoing expert guidance to assist schools throughout the implementation process.

How does Maths — No Problem! support whānau engagement?

Maths — No Problem! provides parent guides and resources to help whānau understand and support their child's learning. We also offer suggestions for home activities that reinforce classroom learning and encourage family involvement.

How does Maths — No Problem! support students with additional learning needs (SEN)?

The programme offers strategies for diverse learners, including those with additional needs. For example, the CPA (Concrete, Pictorial, Abstract) approach benefits students who struggle with traditional methods.

Is there support for ESOL students?

Your ESOL students will benefit from our visual approach and focus on mathematical vocabulary. Your teachers will get strategies to support language development, helping your students build mathematical literacy alongside numeracy skills.

What is maths mastery and how is the approach supported?

Maths mastery develops deep conceptual understanding. This is supported through sequenced lessons, problem-solving focus, and progression from concrete to pictorial to abstract concepts.

Is Maths — No Problem! aligned with Te Mātaiaho?

The programme is specifically tailored to align with Te Mātaiaho, the New Zealand curriculum, covering all required content areas and supporting the development of key competencies outlined in the curriculum.

Still Not Sure?

Join one of our online information sessions..

Sessions run daily Monday - Friday starting at 3:30pm (NZDT)

Let us know if you have any questions and we'll get back to you within 24 hours.

By clicking “Accept All” , you agree to the storing of cookies on your device to enhance site navigation, analyze site usage and assist in our marketing efforts.

Similar Problems from Web Search